Difference between descriptive and inferential statistics
The Descriptive statistics It is the set of statistical methods that describe and / or characterize a group of data. The inferential statistics seeks to deduce and draw conclusions about general situations beyond the set of data obtained.
Statistics is a discipline that is responsible for processing and organizing data, the data being any measure or value that can be obtained through experiments, surveys, censuses or other media. The analysis of the data usually begins with the application of descriptive statistics methods, and then continues with inferential statistics methods.
| Descriptive statistics | Inferential statistics | |
|---|---|---|
| Definition | Methods used to summarize the key characteristics of known data. | Methods that involve the use of sample data to make generalizations or inferences about a population. |
| goals |
Characterize a data group Examine trends or distributions |
Examine differences between groups. Examine if variables are associated. Compare averages between groups. Predict one variable from another. |
| Analysis methods |
Measures of central tendency:
Measures of variability:
|
|
| Application areas | Natural and social sciences |
Social and natural sciences |
| Examples |
|
|
What is descriptive statistics?
The Descriptive statistics It is the part of the statistics that arranges the data so that they can be analyzed and interpreted. Descriptive statistics methods allow us to:
- Determine the central tendency of a variable: average or arithmetic mean, median or mode.
- Determine the variability of a variable: standard deviation, variance, ranges.
- Determine what the distribution of a variable: frequency histogram, normal distribution.
Descriptive statistics examples
When you want to characterize a group of individuals, you use descriptive statistics. For example, we have the following body temperature data for a group of men and women:
| Men | Women |
|---|---|
| 36,1 | 36,2 |
35,9 |
37,2 |
| 36,0 | 37,3 |
| 36,4 | 37,1 |
| 36,3 | 37,0 |
| 36,7 | 37,2 |
| 36,9 | 36,9 |
| 36,8 | 36,8 |
| 37,2 | 36,4 |
| 37,0 | 37,0 |
As they are presented, we cannot draw any conclusion, but when applying descriptive statistics techniques, we can then say that:
- the men in this group have an average temperature of 36.53ºC with a standard deviation of 0.45;
- women in this group have an average temperature of 36.91 ºC, with a standard deviation of 0.36.

What is inferential statistics?
The inferential statistics o statistical inference is the part of statistics that seeks to predict or deduce characteristics or expected results of a population, based on data obtained from a sample of that population. Among the techniques applied in inferential statistics there are:
- The t test: It is used to compare the arithmetic mean of two groups by determining whether the differences between the groups occur randomly or systematically indicating a real difference.
- Analysis of variance or ANOVA: applied to compare two or more groups of variables.
- The correlation analysis: reveals whether the values between two variables tend to change systematically. To make these determinations, the correlation coefficient is used r and the value of p or CI confidence interval.
- Regression analysis: Predict one value from another.
Examples of inferential statistics
If we want to determine if any behavior or biological state is associated with a disease, we use inferential statistical methods. For example, in a study conducted in Germany, 3109 people were evaluated for different health parameters for almost seven years. The results showed that high blood glucose levels (greater than 126 mg / dl fasting), smoking and physical inactivity were associated with the development of dementia.
When a new drug is discovered and its effectiveness is to be demonstrated in a certain disease, inferential statistics are used. In this case, the effects of one group treated with the drug and another group treated with a placebo or a control drug are compared.
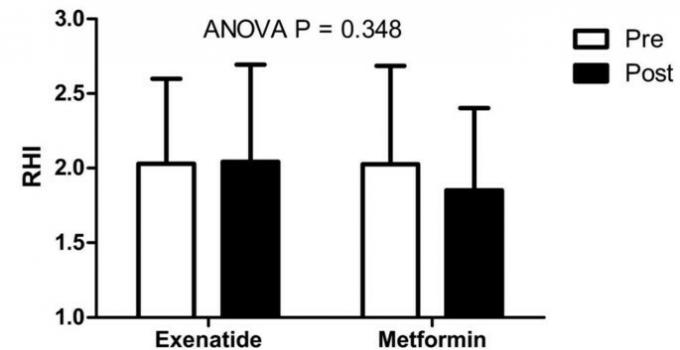
Kelly et al's group investigated endothelial function in 50 obese individuals before (Pre) and after (Post) three months of treatment with two drugs: exenatide and metformin (control). When analyzing the results with the ANOVA technique (P = 0.348; inferential statistics) found that exenatide had no effect on endothelial function, compared to metformin.
References
Hessler, J.B., Ander, K-H, Brönner, M. et al. (2016) Predicting dementia in primary care patients with a cardiovascular health metric: a prospective population-based study. BMC Neurol. 16: 116.
Kelly, A.S., Bergenstal, R.M., Gonzalez-Campoy, J.M., Katz, H., Bank, A.J. (2012) Effects of Exenatide vs Metformin on endothelial function in obese patients with pre-diabetes: a randomized trial. Cardiovascular Diabetology 11:64.



