How to remove the AREA of a PENTAGON
In a Professor we are going to deal with a basic topic for the knowledge of geometry, specifically how to find the area of a pentagon. To do this, we will remember what the area is and what a pentagon is, so that we can then see how to calculate the area of this figure. At the end of the lesson you will find a exercise to practice and, after him, his solution, so that you can check if you have correctly understood what is explained in this lesson.
A pentagon is a five-sided figure any. However, in this article, when we talk about pentagon we will be referring to a polygon of five sides regular, that is, it has its sides of equal length and, therefore, the five angles are equal among them.
These angles measure 108º inside the pentagon, so the sum of the interior angles must be 540º. It also has five vertices, from which we take the diagonals, which end up forming a five-pointed star.
For easily identify itYou can think of a pentagon as a little house. The base will be the floor, the two sides on the left and the right the walls and the upper sides the roof.
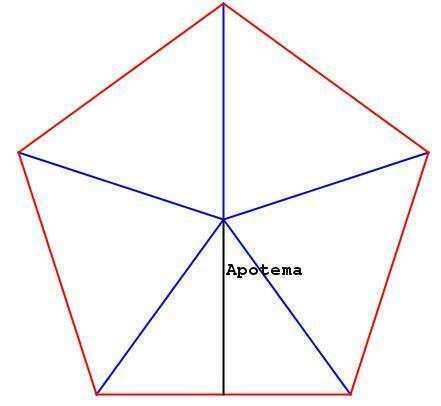
Image: ICT Resources
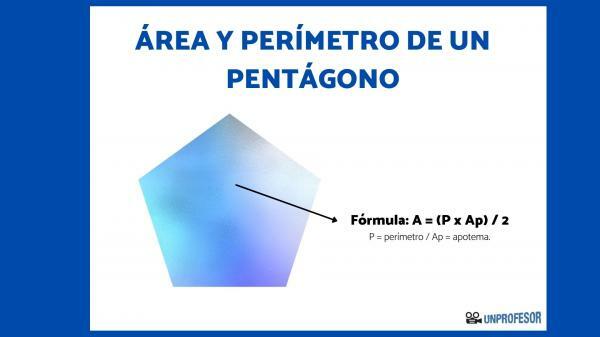
Before we begin to calculate the area of a pentagon, let's remember that the area is the space that a polygon occupies, so it will be in squared units, such as meters squared. To do this, we need the units to be the same in all parts of the formula. The formula is as follows:
A = (P x Ap) / 2
Where P = perimeter and Ap = apothem.
As you can see, new concepts appear to be able to calculate the area. First, the perimeter is nothing more than the sum of all the sides of the pentagon, that is, multiplying one side by 5.
Second, the apothem is calculated from Pythagoras theoremSince a regular pentagon is 5 equilateral triangles joined at a vertex, so if we split each of them in half, we get 10 right triangles. One will suffice: the length of one side will be the hypotenuse, while half of one side will be a leg. The other leg will be the apothem.
Let's look at an example. If we want to calculate the area of a regular pentagon with a side of 15 centimeters, we will need the perimeter, which will be 15 x 5 = 75 cm.
We calculate the apothem with the Pythagorean Theorem: 152 = 7,52 + Ap2; 225 = 56.25 + Ap2; 225 - 56.25 = Ap2; 168.75 = Ap2; Ap = 13 cm. Therefore, we already have the perimeter and the apothem, so we apply the formula: (75 x 13) / 2 = 487.5 cm2.
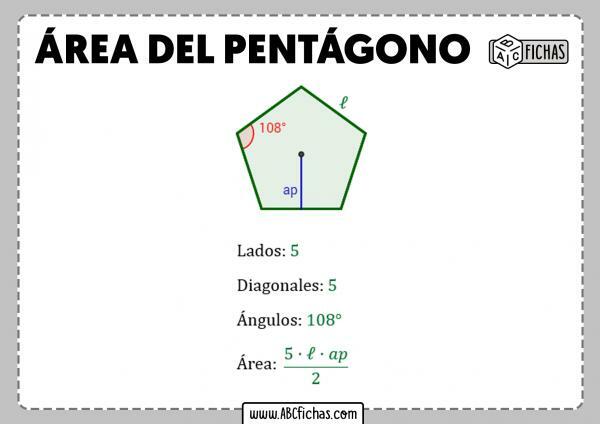
Image: ABC Fichas
To check if you have internalized the concepts, we suggest that you do the following exercises:
- Calculate the area of a regular polygon with five sides of 146 meters perimeter and apothem of 20 meters.
- Find the area of a pentagon 60 centimeters on a side.
Now we will see if you have been able to do the exercises correctly. The answer to the activities is the following:
- We can use the formula directly, since a regular five-sided polygon is a pentagon, so we will multiply the perimeter by the apothem and divide by two: (146 x 20) / 2 = 1460 m2.
- Since we do not have the perimeter or the apothem, we must calculate them first. First, the perimeter will be the sum of the sides, so since it is a pentagon we will have to add 60 five times, so it is easier to multiply 60 by 5, which gives 300. To find out how much the apothem is, we will use Pythagoras as follows: 602 = 302 + Ap2. If we isolate, the apothem gives us 52. Now we can calculate the area: (300 x 52) / 2 = 7800 cm2.
If you found this lesson interesting, feel free to browse the tab Geometry, to find posts similar to this one. On the other hand, we recommend that you use the search engine at the top of the web, so that you can search for everything that is on your mind.

