What is the LAW of SIGNS in mathematics

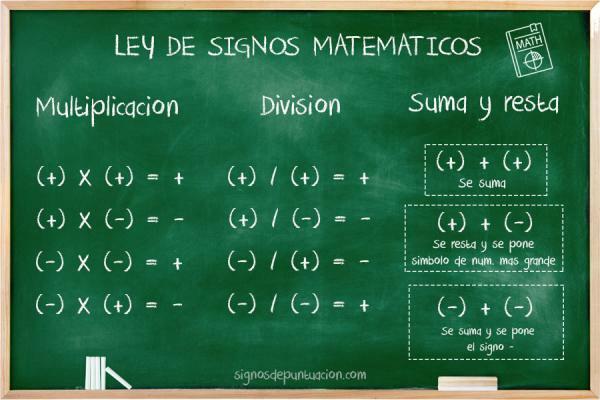
Image: Blendspace

In this math lesson from a Teacher we are going to learn what is the Law of Signs in mathematics. In this way, we will see a section for the law of signs in addition, another for subtraction, a third for multiplication and, finally, a section for division. In addition, throughout the explanation will be added examples so that the law of signs is fully and practically understood. To finish, at the end of the lesson you will be able to practice what you have learned with some exercises and their respective solutions. Ready and prepared for this important lesson?

You may also like: What is a factor in mathematics - with examples

Index

What is the Law of Signs in addition
Law of signs in subtraction
Multiplication with Law of signs and examples
Division with Law of signs and examples
Examples of addition with law of signs
Examples of subtraction with Law of signs
Exercises of the law of signs in mathematics
Solution

What is the Law of Signs in addition.

The addition It is the first operation we learn to do when we start school, but it is essential for the rest of our lives. Also, not only can we add positive numbers, we can also add negative numbers.

instagram story viewer

This is better understood by seeing each of the cases, so:

Yes both numbers are positive, we add the numbers and we get a positive result.
If a number is ppositive and the other negative, we subtract the largest (in absolute value, that is, without taking into account the sign) minus the smallest and the result will be positive or negative, depending on the sign of the largest number.
If both numbers are negative, we add the numbers regardless of their sign, but in the result we keep that negative sign.

Law of signs in subtraction.

We continue to know what the Law of Signs is in mathematics to talk now about the subtraction. It is the operation that we learn after addition and, as in the latter, we can not only subtract positive numbers, we can also subtract negative numbers.

Let's also see it case by case:

If both numbers are positive, the second (the one after the minus sign) will become negative, so we'll get one positive and one negative number, so we will have to subtract the largest (in absolute value, without taking into account the sign) minus the smallest and, as a result, we will have the sign of the number that be older.
If the first number is positive and the second is negative, the one after the subtraction sign, that is, the second one, will become positive, so we will have two positive numbers that we must add and we will have a positive result.
If the first number is negative and the second is positive, the one after the subtraction sign (the second) will become negative, and then what we will do is add the two numbers and the result will be negative.
If both numbers are negative, The one after the sign of the subtraction will become positive and what we will have to do is subtract the largest (in absolute value) minus the smallest and the result will have the sign of the largest.

Multiplication with Law of signs and examples.

Third, the multiplications are very simple operations to do as far as signs are concerned, because the rules that follow are very simple, as you will see below:

If both numbers are positive, We multiply them without taking into account the signs and, once we have the result, we will put a positive sign.
If one number is positive and the other number is negative, we multiply them without taking into account the signs and the result will be negative. It does not matter if the positive is the first or the second and the same with the negative, that is indifferent.
If both numbers are negative, we multiply them without taking into account the signs and the result will be a positive number.

Basically, if the two numbers that we are going to multiply have the same sign, the result is a positive number, while if they have different signs, the result will be negative.

Examples of the law of signs in multiplication

Let's see some examples:

Two positive numbers: (+3) x (+6) = 3 x 6 = 18, since they are both positive: +18.
The first positive number and the second negative: (+4) x (-3) = 4 x 3 = 12, since one is positive and the other negative: -12.
The first positive number and the second negative: (-7) x (+4) = 7 x 4 = 28, since one is positive and the other negative: -28.
Two negative numbers: (-9) x (-5) = 9 x 5 = 45, since they are both negative: +45.

Division with Law of signs and examples.

Lastly, the divisions These are operations that are normally more difficult to understand, but as far as the signs are concerned, they are very simple, because the rules are the same as in multiplications, as you will now see:

If both numbers are positive, We divide them without taking into account the signs and, once we have the result, we will put a positive sign.
If one number is positive and the other number is negative, we divide them without taking into account the signs and the result will be negative. It does not matter if the positive is the first or the second and the same with the negative, that is indifferent.
If both numbers are negative, we divide them without taking into account the signs and the result will be a positive number.

Basically, if the two numbers that we are going to divide have the same sign, the result is a positive number, while if they have different signs, the result will be negative.

Examples of the Law of signs in division

Let's see some examples:

Two positive numbers: (+12): (+3) = 12: 3 = 4, since both are positive: +4.
The first positive number and the second negative: (+20): (-5) = 20: 5 = 4, since one is positive and the other negative: -4.
The first positive number and the second negative: (-8): (+2) = 8: 2 = 4, since one is positive and the other negative: -4.
Two negative numbers: (-9): (-3) = 9: 3 = 3, since they are both negative: -3.

Examples of addition with law of signs.

For the sums, let's see an example for each of the possible cases that we have mentioned in the corresponding section:

Two positive numbers: (+9) + (+1) = 9 + 1 = 10, since both are positive: +10.
One positive number and the other negative: (+8) + (-2), since the largest is 8, we subtract 8 minus 2, which is 6, and since the largest is 8 and is positive, the sign will be positive: +6.
Another example of a positive and a negative number: (+3) + (-10), since the greater is 10, we subtract 10 minus 3, which is 7 and, since the greater is 10 and is negative, the result will also be negative: -7.
Two numbers are negative: (-4) + (-3), what we do is add them without taking into account the signs, so 4 + 3 is 7, but since they are both negative, the result will be -7.

Examples of subtraction with Law of signs.

let's see now examples of the law of signs in subtraction:

Two positive numbers: (+3) - (+2), the second will become negative, so + 3 - 2 will remain, we subtract the largest (3) minus the smallest (2) and it gives 1 and, since the largest was 3, the result will be positive: +1.
First positive and second negative number: (+7) - (-1) the one after the subtraction sign, that is, the -1 will become positive, so we will have + 7 + 1, which added together gives 8 and the sign will be positive: +8.
First negative and second positive number: (-5) - (+4), the one after the minus sign (+4) will become negative, so we will have - 5 - 4 and, then, what we will do is add the two numbers, which gives 5 + 4 = 9 and the result will be in a negative sign, so it will be -9.
Two negative numbers: (-6) - (-2) the one after the subtraction sign will become positive, so - 6 will remain + 2, we will have to subtract the largest (6) minus the smallest (2), which is 4 and the result will have the sign of the largest, that is: -4.

Exercises of the law of signs in mathematics.

Solve the following activities:

1. Solve the sums:

(+3) + (-2)
(+4) + (+5)

2. Solve the subtractions:

(-5) - (+2)
(+6) - (-1)

3. Solve the multiplications:

(+9) x (-4)
(-3) x (-7)

4. Solve the divisions:

(-30): (-5)
(+8): (-4)

Solution.

The solutions are: