How to get the AREA of a RHOMBOID

In this new lesson that we bring you from a Teacher you are going to learn how to find the area of ​​a rhomboid, knowledge that is fundamental and basic to start in geometry and mathematics in general. To consolidate knowledge, we will start by defining what the area is and what figure is a rhomboid, to later explain how the area is calculated in this case. We will also pose a example with an image, to clarify doubts. At the end of the article you will also find training with their respective solutions.

Index

1. What is the area of ​​a rhomboid
2. How to calculate the area of ​​a rhomboid
3. Exercise on finding the area of ​​a rhomboid
4. Solution

The area is the calculation that allows know the space that a polygon occupies determined.

As in today's lesson we are studying the area of ​​a rhomboid, we will be quantifying the space occupied by that rhomboid. It is necessary to remember that the area is measured in units squared, so the result will be m², cm², mm²...

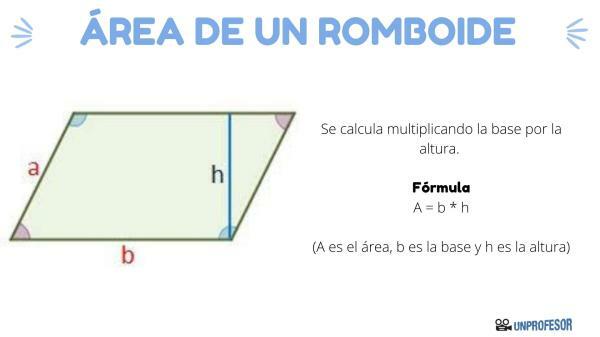
A rhomboid It is a geometric figure that is made up of a parallelogram with four equal sides two by two. That is, it has parallel and equal sides between them two by two (the non-contiguous side). Also, two of its angles are treble and two are obtuse.

To calculate the area of ​​a rhomboid, we will have to follow the following formula:

A = b * h

Where A is the area, b is the base, and h is the height.

We must not confuse the height with the side that is not the base, because the height will go from a vertex of the base perpendicular to the side parallel to the base.

Example

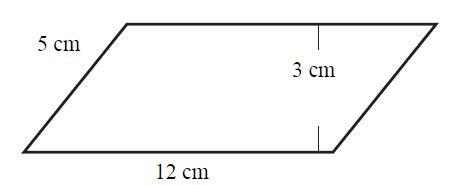
Let's see it better with the example of the image that accompanies this section.

If we have a rhomboid with a base of 12 cm and a side of 5, we only care about the base and the height, which is 3. Thus, the area of ​​this rhomboid will be:

a=b*h=12*3=36cm².

The time has come to put into practice the knowledge acquired in this lesson. We leave you some activities with their response in the following section:

1. Find the area of ​​a rhomboid with base 6 km and height 3 km.

2. Find the area of ​​a rhomboid with base 2 cm and height 30 mm.

3. Justify if the following sentences are true or false:

• A rhomboid has four equal sides.
• A rhomboid has its parallel sides two by two.
• A rhomboid cannot have right angles.

Here we leave the solution to the activities raised in the previous section:

1. Find the area of ​​a rhomboid with base 6 km and height 3 km.

We substitute in the formula and we are left with A = b * h = 6 * 3 = 18 km.

2. Find the area of ​​a rhomboid with base 2 cm and height 30 mm.

As one measurement is in cm and another in mm, we will have to unify them. Since 2 cm is 20 mm, we have a base of 20 mm and a height of 30 mm, so A = b * h = 20 * 30 = 60 mm.

3. Justify if the following sentences are true or false:

• A rhomboid has four equal sides: false, it has its equal sides two by two, unlike, for example, the square, which does have its four equal sides.
• A rhomboid has its parallel sides two by two: true, its opposite sides are parallel.
• A rhomboid cannot have right angles: true, because if it had any right angle it would imply that the sides of that angle were perpendicular, and since they have to be parallel to their opposite side, we would get a square instead of a square. rhomboid. As explained in this lesson, the rhomboids have two acute angles and two obtuse angles, since it is an oblique figure.

If you have come this far it is because you consider this lesson to be useful, so if you want to find more articles on mathematics that are useful to you, you just have to use the search engine at the top of the page Web.

If you want to read more articles similar to How to find the area of ​​a rhomboid, we recommend that you enter our category of Geometry.