How to get the PERIMETER of a scalene triangle

The formula to find the perimeter of a scalene triangle is: P = a + b + c. In unProfesor we explain it to you easily and with examples.

In a new lesson from a Teacher we are going to see how to find the perimeter of a scalene triangle. We will start with the definition of a triangle, then we will continue with the types of triangles that exist to continue with the perimeter of the scalene triangle. Finally, we will see an example of how to find the perimeter of a scalene triangle.

Index

1. Steps to find the perimeter of a scalene triangle - with examples
2. What are scalene triangles: easy definition
3. Characteristics of the triangles
4. types of triangles

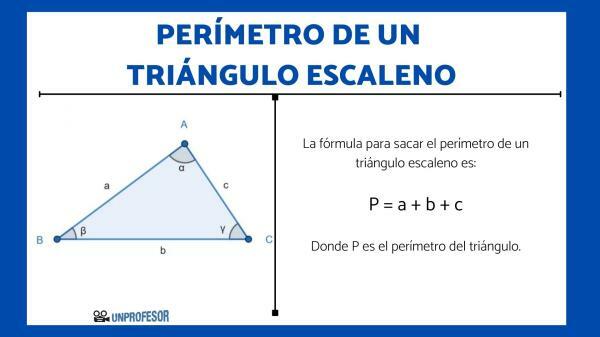
He perimeter is the measure of the length of a figure, that is, the sum of the measure of its contour. In the case of triangles, the perimeter will be the sum of the measure of its three sides.

When we want to calculate the perimeter of a

The formula to find the perimeter of a scalene triangle is:

P = a + b + c

where P is the perimeter of the triangle.

examples

Let's see an example of how to find the perimeter of a scalene triangle.

be a scalene triangle with meassures:

• w = 6cm
• b = 7cm
• c = 4cm

To calculate the perimeter we use the formula seen before

• P = a + b + c
• P = 6 + 7 + 4
• D = 17cm

So the perimeter of the triangle is 17 cm

Let be a scalene triangle of measurements:

• w = 10cm
• b = 8cm
• c = 13cm

To calculate the perimeter we use the formula seen before

• P = a + b + c
• P = 10 + 8 + 13
• D = 31cm

So the perimeter of the triangle is 31 cm

In unProfesor we also tell you how to find the area of ​​a scalene triangle and

The scalene triangles are those who have the measure of its sides are ALL different, that is, none of its sides has the same length.

From this, we can deduce that none of its interior angles will have the same amplitude, meaning that its angles will also all be different.

Depending on the measure of their sides and the amplitude of their angles, scalene triangles can cbe classified into different types:

• Right scalene triangle: They are those triangles that have all their sides unequal but one of its interior angles is right, that is, it measures exactly 90° sexagesimals. The two remaining angles, therefore, will measure less than 90° so they will be acute.
• Acute scalene triangle: are those triangles that have their three interior angles less than 90° sexagesimal, that is, the three angles are acute.
• Obtuse scalene triangle: are those triangles in which the opening of one of its angles is greater than 90° sexagesimal, that is, it is an obtuse angle. While the other two angles are acute.

The triangles, in mathematics, are polygons made up of three sides, three angles, and three vertices. Within geometry, they are the simplest figures after the line. They are considered the most essential figures since any other polygon can be formed from them. That is, polygons can be formed with a sum of triangles. In other words, polygons, by drawing the diagonals, can be decomposed into triangles.

One of the most important characteristics that triangles have is that the sum of their internal angles ALWAYS adds up to 180° sexagesimals.

The sides of a triangle are lines that meet at a point called the vertex. The union of the sides at the vertices form an opening that gives rise to the interior and exterior angles of every triangle.

The triangle characteristicsthey are:

• 3 sided polygon
• its sides meet at vertices
• have 3 vertices
• have 3 interior angles and 3 exterior angles
• The sum of the interior angles always measures 180° sexagesimals.
• is the figure that makes up other polygons

Triangles can be classified according to the measure of its sides wave opening of its angles.

According to the length of its sides

• equilateral triangles: are those that have the length of their three equal sides. That is, the measure of each of its sides is identical, therefore the opening of its interior angles is always 60° sexagesimals each. We can call these rectangles regular polygons.
• isosceles triangles: are those that have the length of two of their equal sides, while the third is different. With this, we can ensure that two of its interior angles will also be equal, while the third will be different.
• scalene triangles: are those that have the length of their three different sides. From what we can tell, its three interior angles will also all be different.

According to the opening of its angles

• right triangles: are those that have one of their angles exactly 90° sexagesimal. That is, one of its angles is right, while the other two are acute. The sides that form the 90° angle are called legs, while the side opposite it is called the hypotenuse.
• oblique triangles: are those that do NOT have any of their right angles. That is, none of its angles measures exactly 90° sexagesimals. Within this classification we find two types of triangles:
• Acute triangles: are those that have their three interior angles of less than 90° sexagesimal, that is, the three angles are acute.
• obtuse triangles: are those that have one of its angles greater than 90° sexagesimal, that is, one of its sides is obtuse, while the other two are acute.

If you want to read more articles similar to How to find the perimeter of a scalene triangle, we recommend that you enter our category of Geometry.